Fuzzy Optimization Design and Research of Cylindrical Coil Spring

Fuzzy Optimization Design and Research of Cylindrical Coil Springs Zhang E Wang Yajun Yan Yanhui Xi'an Jiaotong University, Lean 749 solved the model. The overall optimal structural design scheme can be obtained, so that the spring has the highest reliability and the lightest weight under the condition of bearing capacity and strength. The design example is given in the paper. In the field of mechanical design, there are many uncertain phenomena. Mainly random and fuzzy 1. They are inherently objective attributes. In mechanical reliability analysis and optimization design. Usually only the randomness of variable events is considered, and their ambiguity is ignored. In fact. Many of the variables in the design are a school paste 1 for example. In the strength criterion. Persuasion should be a vague concept. Usually, we specify the spring material 50, the steel's shear fatigue, the strength is 1 = 4 just; 1. According to this, set. 43 is used, but in fact there is no substantial difference between the two. In fact, there is an intermediate transition between full use and complete use. When considering this process. The allowable stress becomes a fuzzy variable. Its boundary is a fuzzy boundary. An effective way to solve the ambiguity problem that exists in engineering is to use fuzzy set theory. This fuzzy variable can be used with fuzzy sets and membership functions. For a long time, in mechanical optimization design, due to the neglect of the objective existence of ambiguity, The design variables and objective functions can't reach the range of values ​​that should be expected, and often miss the true field to reflect the objective attributes of design parameters, which is undoubtedly of great significance and obvious technical and economic benefits for mechanical optimization design. For this reason, a cylindrical coil spring is used as a stone juice object. The optimization of the design of the molding material 1 is more in line with the actual optimization design.

1 Establishment of mathematical model of fuzzy optimization design 1.1 Design example Design the structural size of the valve spring of internal combustion engine. According to the statistics, the parameters of the spring are subject to a normal distribution. The spring material is 500 wire, the shear modulus is load = 750, and the working frequency is 20 out. When the number of cycles, =106, its resistance to shear fatigue, gamma kiss squeaking +, =, for the most human load to open 1; 1.1., =.6 1.2 Design Variables Valve springs are cylindrical helical compression springs that withstand alternating loads, with the exception of materials. In addition to the specified heat treatment conditions, three structural parameters are mainly determined. That is, the diameter of the spring wire 4 spring diameter and the number of work, so the design variable is 1.3 objective function. Since the spring is a key component of the engine valve, high reliability should be the goal. In order to make the spring fuzzy reliability 4 is the highest. The minimum value of the reciprocal can be the objective function when calculating. That is, when only the randomness of the working stress of the spring is considered, and the distribution density function is normal distribution, and the ambiguity of the spring strength is considered, when the membership function is a semi-trapezoidal distribution, according to the fuzzy reliability of mechanical parts The basic theory of design 2 spring work fuzzy reliability is the normal distribution probability density function 扒 is the standard normal distribution function, its value is checked by the normal function; 4 is the mean value of the working stress normal distribution function. The difference is average. Determine the following settings. The maximum shear stress when working for the spring is obtained by the material mechanics 3, which is the shear modulus of the spring material; 7 is the maximum change and the mean and mean square of the stress are the shape; the curvature is considered for processing and manufacturing. The error, usually taken =, 2, =, 57, the production of 0.005 watts into the above formula can be obtained by simplification, and 14 is the membership function of the intensity condition. In particular, the parameters of the semi-trapezoidal distribution 1 are determined by the augmentation coefficient method as follows. 1.4 The condition of the wire diameter of the spring is as follows: the value of the winding ratio is the work of the spring, and the value range is the stability of the spring when the two ends are fixed. At this point, the condition of the resonance-free condition is completed, and the mathematical model of the spring fuzzy optimization design is completed.

2 The basic way to solve the fuzzy optimization problem is to transform it into the conventional optimization. When the mountain method is used to obtain brittleness, the fuzzy optimization model needs to be transformed into the conventional optimization model on the optimal horizontal intercept. The solution process is as follows: Fuzzy comprehensive evaluation to determine the optimal level threshold 1 is now determined to affect 1! The factor factor level and its membership degree 1. The membership value is determined by expert scoring method.

2 Determine the alternative set. The design object of this design is the cut-off level whose value range is 1 interval. According to the design conditions and requirements, the selection set is X=3 to determine the factor weight set. In order to accurately reflect the influence of various factors and factor levels on the judges, the weights of each factor and factor level should be given, and the weights of the factors should be determined according to the design conditions! For the level fuzzy comprehensive evaluation. The fuzzy comprehensive evaluation set is obtained by the fuzzy matrix multiplication rule transformation. .尽=0.60.70.660.37, 0.1. The 芩 is the fuzzy relation matrix obtained by arranging the values ​​of the respective recorded functions in the order of 1.

Determine the assessment results. According to the principle of maximum membership, the optimal level cut-in is 6 after conversion to the conventional optimization model. The complex method is used to solve the modulo 1.26 from the objective function 1 = and, so the fuzzy reliability is obtained and =89879.

That is, when the valve spring is subjected to the alternating load number up to 6, the working reliability can reach 89.

879. The optimization parameter after rounding is 1=4.53557; this structural parameter reduces the spring weight by 13.80 compared with the conventional design. The optimal design angle of the crank slider mechanism is compared with the two design results of the fuzzy optimization design and the conventional design. The design of the Weijie Benxi Metallurgical Specialized Mechanical Engineering Department, the design of the optimal transmission angle of the Benxi 1722 crank slider mechanism has been introduced in many literatures, but most of them introduce the method of investigation or solution. Here, the analytical formula is used to derive the calculation formula, which is more convenient than the former. more acurrate.

1 The crank slider mechanism is designed to be simple if the stroke of the slider is set to 0 = 0.

Take the point on the extension line of the stroke, so that the center of the circle is rounded with the radius of the crank of =572. When the crank and the stroke 5 are vertically up and down, the transmission angle of the mechanism is the smallest, and the pressure angle at this time is the largest. The geometric relationship in 1 can be seen from the gate type, it is better to know the maximum transmission angle at the point; but it also increases the overall size of the mechanism. If given in advance, the influencing factors of the input value and the degree of membership affect the degree of membership degree of manufacturing level 2 material quality and good condition 3 use condition 4 importance degree 5 bureaus are better, better, less important, slightly more important, lower, worse Important low-difference important two design results compared wire diameter mm spring diameter, 1 working circle reliability design results fuzzy optimization results 3 Conclusion 1 The fuzzy optimization design of cylindrical helical springs is considered here, because of the consideration of various design parameters Randomness and ambiguity, and can take advantage of the optimization design, so the designed scheme is more reasonable and more scientific than the objective reality. 2 Example design results The fuzzy optimization design not only makes the spring obtain higher working reliability, but also makes it the lightest, which shows the technical and economic benefits and superiority of fuzzy optimization. 3 The method provided can be applied to the spring design.

Wang Peizhuang. Fuzzy set theory and its applications. Shanghai Shanghai Science and Technology Press, 1993.

Liu Yangsong, Li Wenfang. A fuzzy method of mechanical design. Beijing Machinery Industry Press, Lin Shuqi. Material mechanics. Xi'an Xi'an Jiaotong University Press, 1984.

Stainless Steel Twill Dutch Weave Mesh, this kind of Woven Steel Mesh is weaved by a special weave technology, which integrate the Dutch weave and twill weave.  The thicker warp wire and the thinner weft staggered. Each warp wire shuttles up and down with the weft wire.

Features: Stainless Steel Wire Mesh screen has the advantages of smooth surface, high hardness, constant mesh, no deformation, high temperature resistance, corrosion resistance, flexible network, excellent filtration performance, good filtration accuracy, high load intensity, high temperature resistance, low temperature resistance and so on

Material: SS304, SS 316,SS316L

Use: Stainless Mesh is mainly used for gas, liquid filtration and other medium separation; widely used in precision pressure filter, fuel filter, vacuum filter, filter material, do aerospace, oil refining, pharmaceutical, sugar, oil, chemical industry, chemical fiber, rubber, tire manufacturing, metallurgy, food, health industry and scientific research.

Specification: The standard roll width: 1m-2m. Standard roll length: 30-50m.The steel mesh in special width and length can be produced according to customers` requirements.

Structural drawing:

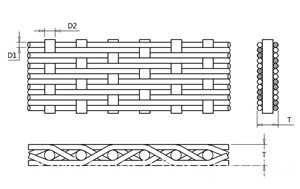
The warp wire (D1) : all longitudinal braided lines.

The weft wire (D2) : all horizontal weaving lines.

Aperture : the distance between two meridians or two weft.

Mesh number: number of mesh holes per inch.

Thickness (T) : thickness of the wire mesh.

Speciation List:

Stainless Steel Twill Dutch Weave Mesh

Stainless Steel Twill Dutch Weave Mesh,Extra Fine Stainless Steel Mesh,Stainless Steel Screen Mesh,Twill Dutch Weave Mesh

Anping Xinzheng Metal Wire Mesh Co., Ltd , https://www.sievingmesh.com